Kuantum faz uzayında sürekli büyükleştirme

Kuantum faz uzayında sürekli büyükleştirme

Zaman Damgası: 24 Mayıs 2023 7:39
Kaynak Düğüm: 2674950

Zacharie Van Herstraeten1,2, Michael G.Jabbour1,3,4ve Nicolas J. Cerf1

1Kuantum Bilgi ve İletişim Merkezi, École polytechnique de Bruxelles, CP 165/59, Université libre de Bruxelles, 1050 Brüksel, Belçika
2Wyant College of Optical Sciences, The University of Arizona, 1630 E. University Blvd., Tucson, AZ 85721, ABD
3DAMTP, Matematik Bilimleri Merkezi, Cambridge Üniversitesi, Cambridge CB3 0WA, Birleşik Krallık
4Fizik Bölümü, Danimarka Teknik Üniversitesi, 2800 Kongens Lyngby, Danimarka

Bu makaleyi ilginç mi buldunuz yoksa tartışmak mı istiyorsunuz? SciRate'e çığlık at veya yorum bırak.

Özet

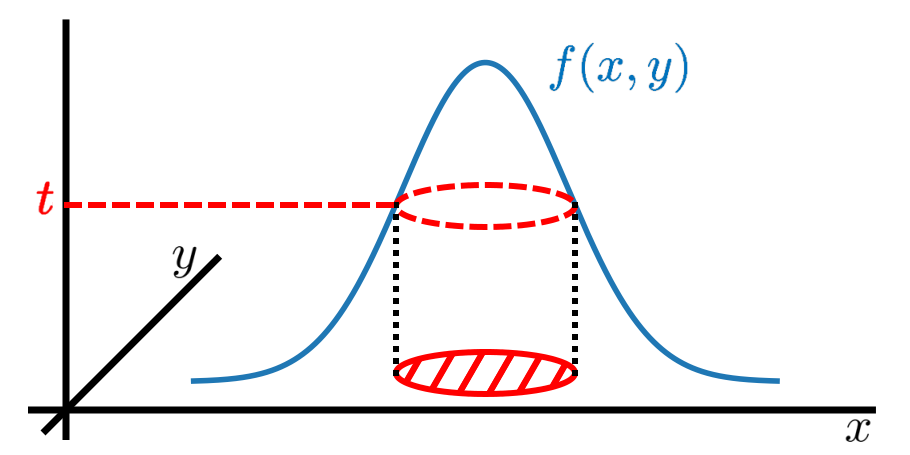
We explore the role of majorization theory in quantum phase space. To this purpose, we restrict ourselves to quantum states with positive Wigner functions and show that the continuous version of majorization theory provides an elegant and very natural approach to exploring the information-theoretic properties of Wigner functions in phase space. After identifying all Gaussian pure states as equivalent in the precise sense of continuous majorization, which can be understood in light of Hudson's theorem, we conjecture a fundamental majorization relation: any positive Wigner function is majorized by the Wigner function of a Gaussian pure state (especially, the bosonic vacuum state or ground state of the harmonic oscillator). As a consequence, any Schur-concave function of the Wigner function is lower bounded by the value it takes for the vacuum state. This implies in turn that the Wigner entropy is lower bounded by its value for the vacuum state, while the converse is notably not true. Our main result is then to prove this fundamental majorization relation for a relevant subset of Wigner-positive quantum states which are mixtures of the three lowest eigenstates of the harmonic oscillator. Beyond that, the conjecture is also supported by numerical evidence. We conclude by discussing some implications of this conjecture in the context of entropic uncertainty relations in phase space.

Popüler özet

Belirsizlik ilkesi, kuantum fiziğinin en büyüleyici fenomenlerinden biridir. Bir parçacığın konumu ve momentumu gibi ölçülebilir nicelik çiftlerinin aynı anda doğru bir şekilde tahmin edilebilmesi doğal görünse de, kuantum fiziği aslında değişmeyen gözlemlenebilirler için bunu yasaklar. Heisenberg ve Kennard, belirsizliğini yakalamak için ölçülebilir herhangi bir miktarın varyansını kullanarak bunu kesinleştirdi. Yıllar sonra, Heisenberg'in belirsizlik ilkesi, belirsizliği ölçmek için uygun bir araç olarak entropiye dönülerek yeniden formüle edildi. Burada, faz uzayındaki kuantum değişkenlerinin belirsizliğini anlamak için daha güçlü bir bilgi-kuramsal paradigma, yani büyükleştirme teorisini tanıtıyoruz.

Bu matematiksel teori, bir asırdan fazla bir süre önce geliştirildi ve istatistikten fiziğe kadar çok sayıda bilim alanında kullanıldı. Dikkat çekici bir şekilde, kuantum fiziğine ancak nispeten yakın bir zamanda uygulandı ve burada kuantum dolaşıklığı keşfetmek için güçlü bir yaklaşım olduğu gösterildi. Bu nedenle, faz uzayındaki kuantum değişkenlerini, yani Wigner fonksiyonlarını tanımlayan sürekli yoğunlukları karakterize etmek için hiçbir zaman kullanılmadı. Bunun için uygun bir araç olarak sürekli büyükleştirme gösteriyoruz. Makalemizin ana itici gücü, bir bosonik modun (yani, harmonik osilatörün temel durumu) vakum durumunun Wigner fonksiyonunun, diğer herhangi bir Wigner fonksiyonunu sürekli olarak büyükleştirdiği ve onu büyükleştirme anlamında daha az belirsiz hale getirdiği ifadesiyle ilgilidir. .

Sonuçlarımızı kuantum optiği bağlamında açıklayıp tartışırken, bunlar herhangi bir kanonik çifte aktarılır ve bu nedenle fiziğin çeşitli alanlarında çıkarımları olmalıdır.

► BibTeX verileri

► Referanslar

[1] G. H. Hardy, J. E. Littlewood, and G. Pólya, ``Inequalities,''. Cambridge University Press, 1934.
https: / / doi.org/ 10.2307 / 3605504

[2] A. W. Marshall, I. Olkin, and B. C. Arnold, ``Inequalities: Theory of Majorization and its Applications,'', vol. 143. Springer, second ed., 2011.
https:/​/​doi.org/​10.1007/​978-0-387-68276-1

[3] T. Ando, ``Majorization, doubly stochastic matrices, and comparison of eigenvalues,'' Linear Algebra Appl. 118, 163–248 (1989).
https:/​/​doi.org/​10.1016/​0024-3795(89)90580-6

[4] K. Mosler, ``Majorization in economic disparity measures,'' Linear Algebra and its Applications 199, 91–114 (1994).
https:/​/​doi.org/​10.1016/​0024-3795(94)90343-3

[5] T. van Erven and P. Harremoës, ``Rényi divergence and majorization,'' in 2010 IEEE International Symposium on Information Theory, pp. 1335–1339, IEEE. 2010.
https: / / doi.org/ 10.1109 / ISIT.2010.5513784

[6] M. A. Alhejji and G. Smith, ``A Tight Uniform Continuity Bound for Equivocation,'' in 2020 IEEE International Symposium on Information Theory (ISIT), pp. 2270–2274. 2020.
https: / / doi.org/ 10.1109 / ISIT44484.2020.9174350

[7] M. G. Jabbour and N. Datta, ``A Tight Uniform Continuity Bound for the Arimoto-Rényi Conditional Entropy and its Extension to Classical-Quantum States,'' IEEE Transactions on Information Theory 68, 2169–2181 (2022).
https: / / doi.org/ 10.1109 / TIT.2022.3142812

[8] A. Horn, ``Doubly Stochastic Matrices and the Diagonal of a Rotation Matrix,'' American Journal of Mathematics 76, 620–630 (1954).
https: / / doi.org/ 10.2307 / 2372705

[9] M. A. Nielsen, ``Conditions for a Class of Entanglement Transformations,'' Physical Review Letters 83, 436 (1999).
https: / / doi.org/ 10.1103 / PhysRevLett.83.436

[10] M. A. Nielsen and G. Vidal, ``Majorization and the interconversion of bipartite states,'' Quantum Information and Computation 1, 76–93 (2001).
https: / / doi.org/ 10.26421 / QIC1.1-5

[11] M. A. Nielsen and J. Kempe, ``Separable States Are More Disordered Globally than Locally,'' Physical Review Letters 86, 5184–5187 (2001).
https: / / doi.org/ 10.1103 / PhysRevLett.86.5184

[12] T. Hiroshima, ``Majorization Criterion for Distillability of a Bipartite Quantum State,'' Physical Review Letters 91, 057902 (2003).
https: / / doi.org/ 10.1103 / PhysRevLett.91.057902

[13] Z. Puchała, Ł. Rudnicki, and K. Życzkowski, ``Majorization entropic uncertainty relations,'' Journal of Physics A: Mathematical and Theoretical 46, 272002 (2013).
https:/​/​doi.org/​10.1088/​1751-8113/​46/​27/​272002

[14] L. Rudnicki, Z. Puchała, and K. Życzkowski, ``Strong majorization entropic uncertainty relations,'' Physical Review A 89, 052115 (2014).
https: / / doi.org/ 10.1103 / PhysRevA.89.052115

[15] L. Rudnicki, ``Majorization approach to entropic uncertainty relations for coarse-grained observables,'' Physical Review A 91, 032123 (2015).
https: / / doi.org/ 10.1103 / PhysRevA.91.032123

[16] F. Brandão, M. Horodecki, N. Ng, J. Oppenheim, and S. Wehner, ``The second laws of quantum thermodynamics,'' Proceedings of the National Academy of Sciences 112, 3275–3279 (2015).
https: / / doi.org/ 10.1073 / pnas.1411728112

[17] R. García-Patrón, C. Navarrete-Benlloch, S. Lloyd, J. H. Shapiro, and N. J. Cerf, ``Majorization Theory Approach to the Gaussian Channel Minimum Entropy Conjecture,'' Physical Review Letters 108, 110505 (2012).
https: / / doi.org/ 10.1103 / PhysRevLett.108.110505

[18] C. N. Gagatsos, O. Oreshkov, and N. J. Cerf, ``Majorization relations and entanglement generation in a beam splitter,'' Physical Review A 87, 042307 (2013).
https: / / doi.org/ 10.1103 / PhysRevA.87.042307

[19] G. De Palma, D. Trevisan, and V. Giovannetti, ``Passive States Optimize the Output of Bosonic Gaussian Quantum Channels,'' IEEE Transactions on Information Theory 62, 2895–2906 (2016).
https: / / doi.org/ 10.1109 / TIT.2016.2547426

[20] M. G. Jabbour, R. García-Patrón, and N. J. Cerf, ``Majorization preservation of Gaussian bosonic channels,'' New Journal of Physics 18, 073047 (2016).
https:/​/​doi.org/​10.1088/​1367-2630/​18/​7/​073047

[21] M. G. Jabbour and N. J. Cerf, ``Fock majorization in bosonic quantum channels with a passive environment,'' Journal of Physics A: Mathematical and Theoretical 52, 105302 (2019).
https:/​/​doi.org/​10.1088/​1751-8121/​aaf0d2

[22] U. Leonhardt, ``Essential quantum optics: from quantum measurements to black holes,''. Cambridge University Press, 2010.
https: / / doi.org/ 10.1017 / CBO9780511806117

[23] A. Hertz, M. G. Jabbour, and N. J. Cerf, ``Entropy-power uncertainty relations: towards a tight inequality for all Gaussian pure states,'' Journal of Physics A: Mathematical and Theoretical 50, 385301 (2017).
https: / / doi.org/ 10.1088 / 1751-8121 / aa852f

[24] A. Hertz and N. J. Cerf, ``Continuous-variable entropic uncertainty relations,'' Journal of Physics A: Mathematical and Theoretical 52, 173001 (2019).
https:/​/​doi.org/​10.1088/​1751-8121/​ab03f3

[25] C. Weedbrook, S. Pirandola, R. García-Patrón, N. J. Cerf, T. C. Ralph, J. H. Shapiro, and S. Lloyd, ``Gaussian quantum information,'' Review of Modern Physics 84, 621–669 (2012).
https: / / doi.org/ 10.1103 / RevModPhys.84.621

[26] Z. Van Herstraeten and N. J. Cerf, ``Quantum Wigner entropy,'' Physical Review A 104, 042211 (2021).
https: / / doi.org/ 10.1103 / PhysRevA.104.042211

[27] F. J. Narcowich, ``Distributions of $hbar$-positive type and applications,'' Journal of mathematical physics 30, 2565–2573 (1989).
https: / / doi.org/ 10.1063 / 1.528537

[28] T. Bröcker and R. Werner, ``Mixed states with positive Wigner functions,'' Journal of mathematical physics 36, 62–75 (1995).
https: / / doi.org/ 10.1063 / 1.531326

[29] R. L. Hudson, ``When is the Wigner quasi-probability density non-negative?,'' Reports on Mathematical Physics 6, 249–252 (1974).
https:/​/​doi.org/​10.1016/​0034-4877(74)90007-X

[30] F. Soto and P. Claverie, ``When is the Wigner function of multidimensional systems nonnegative?,'' Journal of Mathematical Physics 24, 97–100 (1983).
https: / / doi.org/ 10.1063 / 1.525607

[31] F. J. Narcowich and R. O’Connell, ``Necessary and sufficient conditions for a phase-space function to be a Wigner distribution,'' Physical Review A 34, 1 (1986).
https: / / doi.org/ 10.1103 / PhysRevA.34.1

[32] A. Mandilara, E. Karpov, and N. J. Cerf, ``Extending Hudson's theorem to mixed quantum states,'' Physical Review A 79, 062302 (2009).
https: / / doi.org/ 10.1103 / PhysRevA.79.062302

[33] A. Mandilara, E. Karpov, and N. Cerf, ``Gaussianity bounds for quantum mixed states with a positive Wigner function,'' in Journal of Physics: Conference Series, vol. 254, p. 012011, IOP Publishing. 2010.
https:/​/​doi.org/​10.1088/​1742-6596/​254/​1/​012011

[34] L. Wang and M. Madiman, ``Beyond the Entropy Power Inequality, via Rearrangements,'' IEEE Transactions on Information Theory 60, 5116–5137 (2014).
https: / / doi.org/ 10.1109 / TIT.2014.2338852

[35] G. H. Hardy, J. E. Littlewood, and G. Pólya, ``Some simple inequalities satisfied by convex functions,'' Messenger of Mathematics 58, 145–152 (1929).

[36] H. Joe, ``An ordering of dependence for distribution of k-tuples, with applications to lotto games,'' Canadian Journal of Statistics 15, 227–238 (1987).
https: / / doi.org/ 10.2307 / 3314913

[37] I. Schur, ``Uber eine Klasse von Mittelbildungen mit Anwendungen die Determinanten,'' Sitzungsberichte der Berliner Mathematischen Gesellschaft 22, 416–427 (1923).

[38] A. W. Roberts and D. E. Varberg, ``Convex functions,''. Academic Press New York, 1973.
https:/​/​doi.org/​10.1016/​B978-0-444-89597-4.50013-5

[39] A. Rényi, ``On measures of entropy and information,'' in Proceedings of the Fourth Berkeley Symposium on Mathematical Statistics and Probability, Volume 1: Contributions to the Theory of Statistics, vol. 4, pp. 547–562, University of California Press. 1961.

[40] Y. He, A. B. Hamza, and H. Krim, ``A generalized divergence measure for robust image registration,'' IEEE Transactions on Signal Processing 51, 1211–1220 (2003).
https://​/​doi.org/​10.1109/​TSP.2003.810305

[41] J. V. Ryff, ``Orbits of $L^1$-functions under doubly stochastic transformations,'' Transactions of the American Mathematical Society 117, 92–100 (1965).
https: / / doi.org/ 10.2307 / 1994198

[42] F. Bahrami, S. M. Manjegani, and S. Moein, ``Semi-doubly Stochastic Operators and Majorization of Integrable Functions,'' Bulletin of the Malaysian Mathematical Sciences Society 44, 693–703 (2021).
https:/​/​doi.org/​10.1007/​s40840-020-00971-2

[43] S. M. Manjegani and S. Moein, ``Majorization and semidoubly stochastic operators on $ L^{1}(X)$,'' Journal of Inequalities and Applications 2023, 1–20 (2023).
HTTPS: / / doi.org/ 10.1186 / s13660-023-02935-z

[44] I. Białynicki-Birula and J. Mycielski, ``Uncertainty relations for information entropy in wave mechanics,'' Communications in Mathematical Physics 44, 129–132 (1975).
https: / / doi.org/ 10.1007 / BF01608825

[45] A. Wehrl, ``General properties of entropy,'' Reviews of Modern Physics 50, 221 (1978).
https: / / doi.org/ 10.1103 / RevModPhys.50.221

[46] E. H. Lieb, ``Proof of an entropy conjecture of Wehrl,'' in Inequalities, pp. 359–365. Springer, 2002.
https:/​/​doi.org/​10.1007/​978-3-642-55925-9_30

[47] E. H. Lieb and J. P. Solovej, ``Proof of an entropy conjecture for Bloch coherent spin states and its generalizations,'' Acta Mathematica 212, 379 (2014).
https:/​/​doi.org/​10.1007/​s11511-014-0113-6

[48] J. R. Johansson, P. D. Nation, and F. Nori, ``QuTiP: An open-source Python framework for the dynamics of open quantum systems,'' Computer Physics Communications 183, 1760–1772 (2012).
https: / / doi.org/ 10.1016 / j.cpc.2012.02.021

[49] K. Życzkowski, P. Horodecki, A. Sanpera, and M. Lewenstein, ``Volume of the set of separable states,'' Physical Review A 58, 883 (1998).
https: / / doi.org/ 10.1103 / PhysRevA.58.883

Alıntılama

[1] Nuno Costa Dias and João Nuno Prata, "On a recent conjecture by Z. Van Herstraeten and N.J. Cerf for the quantum Wigner entropy", arXiv: 2303.10531, (2023).

[2] Zacharie Van Herstraeten and Nicolas J. Cerf, "Quantum Wigner entropy", Fiziksel İnceleme A 104 4, 042211 (2021).

[3] Martin Gärttner, Tobias Haas, and Johannes Noll, "Detecting continuous variable entanglement in phase space with the $Q$-distribution", arXiv: 2211.17165, (2022).

Yukarıdaki alıntılar SAO / NASA REKLAMLARI (son başarıyla 2023-05-24 23:55:18) güncellendi. Tüm yayıncılar uygun ve eksiksiz alıntı verisi sağlamadığından liste eksik olabilir.

On Crossref'in alıntı hizmeti alıntı yapma çalışmaları ile ilgili veri bulunamadı (son deneme 2023-05-24 23:55:17).

Bu Makale, Quantum'da Creative Commons Atıf 4.0 Uluslararası (CC BY 4.0) lisans. Telif hakkı, yazarlar veya kurumları gibi orijinal telif hakkı sahiplerine aittir.

Zaman Damgası:

Den fazla Kuantum Günlüğü