Continuous Majorization In Quantum Phase Space

เผยแพร่ซ้ำโดยเพลโต

ผู้ติดตาม: 0

ซาคารี ฟาน เฮอร์สตราเทน^1,2, ไมเคิล จี. แจ็บเบอร์^1,3,4และ Nicolas J. Cerf¹

¹ศูนย์ข้อมูลควอนตัมและการสื่อสาร École polytechnique de Bruxelles, CP 165/59, Université libre de Bruxelles, 1050 Brussels, Belgium
²Wyant College of Optical Sciences, University of Arizona, 1630 E. University Blvd., Tucson, AZ 85721, USA
³DAMTP ศูนย์วิทยาศาสตร์คณิตศาสตร์ มหาวิทยาลัยเคมบริดจ์ Cambridge CB3 0WA สหราชอาณาจักร
⁴ภาควิชาฟิสิกส์ มหาวิทยาลัยเทคนิคแห่งเดนมาร์ก 2800 Kongens Lyngby ประเทศเดนมาร์ก

พบบทความนี้ที่น่าสนใจหรือต้องการหารือ? Scite หรือแสดงความคิดเห็นใน SciRate.

นามธรรม

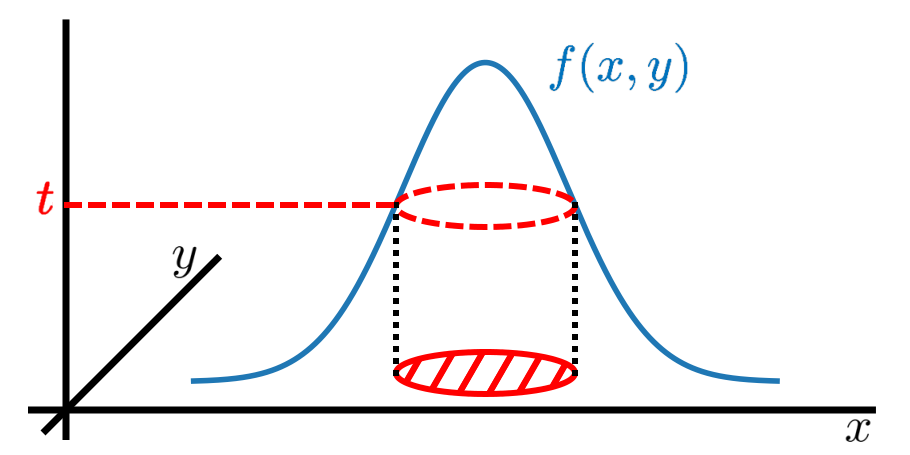
We explore the role of majorization theory in quantum phase space. To this purpose, we restrict ourselves to quantum states with positive Wigner functions and show that the continuous version of majorization theory provides an elegant and very natural approach to exploring the information-theoretic properties of Wigner functions in phase space. After identifying all Gaussian pure states as equivalent in the precise sense of continuous majorization, which can be understood in light of Hudson's theorem, we conjecture a fundamental majorization relation: any positive Wigner function is majorized by the Wigner function of a Gaussian pure state (especially, the bosonic vacuum state or ground state of the harmonic oscillator). As a consequence, any Schur-concave function of the Wigner function is lower bounded by the value it takes for the vacuum state. This implies in turn that the Wigner entropy is lower bounded by its value for the vacuum state, while the converse is notably not true. Our main result is then to prove this fundamental majorization relation for a relevant subset of Wigner-positive quantum states which are mixtures of the three lowest eigenstates of the harmonic oscillator. Beyond that, the conjecture is also supported by numerical evidence. We conclude by discussing some implications of this conjecture in the context of entropic uncertainty relations in phase space.

สรุปยอดนิยม

หลักการความไม่แน่นอนเป็นหนึ่งในปรากฏการณ์ที่น่าสนใจที่สุดในฟิสิกส์ควอนตัม แม้ว่าอาจดูเหมือนเป็นธรรมชาติที่คู่ของปริมาณที่วัดได้ เช่น ตำแหน่งและโมเมนตัมของอนุภาค สามารถทำนายได้อย่างแม่นยำพร้อมๆ กัน แต่จริงๆ แล้วฟิสิกส์ควอนตัมห้ามสิ่งนี้ไว้สำหรับสิ่งสังเกตที่ไม่ได้เคลื่อนที่ ไฮเซนเบิร์กและเคนนาร์ดทำสิ่งนี้ได้อย่างแม่นยำโดยใช้ความแปรปรวนของปริมาณที่วัดได้เพื่อจับความไม่แน่นอน หลายปีต่อมา หลักการความไม่แน่นอนของไฮเซนเบิร์กได้รับการปรับปรุงใหม่โดยเปลี่ยนมาใช้เอนโทรปีเป็นวิธีการที่เหมาะสมในการหาปริมาณความไม่แน่นอน ที่นี่ เราแนะนำกระบวนทัศน์เชิงทฤษฎีข้อมูลที่แข็งแกร่งยิ่งขึ้นสำหรับการทำความเข้าใจความไม่แน่นอนของตัวแปรควอนตัมในเฟสสเปซ ซึ่งก็คือทฤษฎีการแบ่งกลุ่มเป็นสำคัญ

ทฤษฎีทางคณิตศาสตร์นี้ได้รับการพัฒนามากว่าหนึ่งศตวรรษแล้ว และถูกนำมาใช้ในสาขาวิทยาศาสตร์มากมาย ตั้งแต่สถิติไปจนถึงฟิสิกส์ ที่น่าทึ่งคือมันถูกนำไปใช้กับฟิสิกส์ควอนตัมเมื่อไม่นานมานี้ ซึ่งแสดงให้เห็นว่าเป็นแนวทางที่ทรงพลังสำหรับการสำรวจความพัวพันของควอนตัม ด้วยเหตุนี้ จึงไม่เคยถูกนำมาใช้เพื่อระบุลักษณะความหนาแน่นต่อเนื่องที่อธิบายตัวแปรควอนตัมในเฟสสเปซ นั่นคือ ฟังก์ชันวิกเนอร์ เราแสดงวิชาเอกอย่างต่อเนื่องเพื่อเป็นเครื่องมือที่เหมาะสมสำหรับสิ่งนี้ แรงผลักดันหลักของบทความของเราเกี่ยวข้องกับข้อความที่ว่าฟังก์ชัน Wigner ของสถานะสุญญากาศของโหมดบอสโซนิก (เช่น สถานะกราวด์ของออสซิลเลเตอร์ฮาร์มอนิก) ขยายฟังก์ชัน Wigner อื่นๆ อย่างต่อเนื่อง ทำให้มีความไม่แน่นอนน้อยลงในแง่ของการแปรเป็นสำคัญ .

ในขณะที่เราเปิดเผยและหารือเกี่ยวกับผลลัพธ์ของเราในบริบทของควอนตัมออปติก สิ่งเหล่านี้จะส่งต่อไปยังคู่ที่ยอมรับได้ ดังนั้นควรมีความหมายโดยนัยในด้านต่างๆ ของฟิสิกส์

► ข้อมูล BibTeX

@article{VanHerstraeten2023continuous, doi = {10.22331/q-2023-05-24-1021}, url = {https://doi.org/10.22331/q-2023-05-24-1021}, title = {Continuous majorization in quantum phase space}, author = {Van Herstraeten, Zacharie and Jabbour, Michael G. and Cerf, Nicolas J.}, journal = {{Quantum}}, issn = {2521-327X}, publisher = {{Verein zur F{"{o}}rderung des Open Access Publizierens in den Quantenwissenschaften}}, volume = {7}, pages = {1021}, month = may, year = {2023}
}

► ข้อมูลอ้างอิง

[1] G. H. Hardy, J. E. Littlewood, and G. Pólya, ``Inequalities,''. Cambridge University Press, 1934.
https://doi.org/10.2307/3605504

[2] A. W. Marshall, I. Olkin, and B. C. Arnold, ``Inequalities: Theory of Majorization and its Applications,'', vol. 143. Springer, second ed., 2011.
https://doi.org/10.1007/978-0-387-68276-1

[3] T. Ando, ``Majorization, doubly stochastic matrices, and comparison of eigenvalues,'' Linear Algebra Appl. 118, 163–248 (1989).
https://doi.org/10.1016/0024-3795(89)90580-6

[4] K. Mosler, ``Majorization in economic disparity measures,'' Linear Algebra and its Applications 199, 91–114 (1994).
https://doi.org/10.1016/0024-3795(94)90343-3

[5] T. van Erven and P. Harremoës, ``Rényi divergence and majorization,'' in 2010 IEEE International Symposium on Information Theory, pp. 1335–1339, IEEE. 2010.
https://doi.org/10.1109/ISIT.2010.5513784

[6] M. A. Alhejji and G. Smith, ``A Tight Uniform Continuity Bound for Equivocation,'' in 2020 IEEE International Symposium on Information Theory (ISIT), pp. 2270–2274. 2020.
https://doi.org/10.1109/ISIT44484.2020.9174350

[7] M. G. Jabbour and N. Datta, ``A Tight Uniform Continuity Bound for the Arimoto-Rényi Conditional Entropy and its Extension to Classical-Quantum States,'' IEEE Transactions on Information Theory 68, 2169–2181 (2022).
https://doi.org/10.1109/TIT.2022.3142812

[8] A. Horn, ``Doubly Stochastic Matrices and the Diagonal of a Rotation Matrix,'' American Journal of Mathematics 76, 620–630 (1954).
https://doi.org/10.2307/2372705

[9] M. A. Nielsen, ``Conditions for a Class of Entanglement Transformations,'' Physical Review Letters 83, 436 (1999).
https://doi.org/10.1103/PhysRevLett.83.436

[10] M. A. Nielsen and G. Vidal, ``Majorization and the interconversion of bipartite states,'' Quantum Information and Computation 1, 76–93 (2001).
https://doi.org/10.26421/QIC1.1-5

[11] M. A. Nielsen and J. Kempe, ``Separable States Are More Disordered Globally than Locally,'' Physical Review Letters 86, 5184–5187 (2001).
https://doi.org/10.1103/PhysRevLett.86.5184

[12] T. Hiroshima, ``Majorization Criterion for Distillability of a Bipartite Quantum State,'' Physical Review Letters 91, 057902 (2003).
https://doi.org/10.1103/PhysRevLett.91.057902

[13] Z. Puchała, Ł. Rudnicki, and K. Życzkowski, ``Majorization entropic uncertainty relations,'' Journal of Physics A: Mathematical and Theoretical 46, 272002 (2013).
https://doi.org/10.1088/1751-8113/46/27/272002

[14] L. Rudnicki, Z. Puchała, and K. Życzkowski, ``Strong majorization entropic uncertainty relations,'' Physical Review A 89, 052115 (2014).
https://doi.org/10.1103/PhysRevA.89.052115

[15] L. Rudnicki, ``Majorization approach to entropic uncertainty relations for coarse-grained observables,'' Physical Review A 91, 032123 (2015).
https://doi.org/10.1103/PhysRevA.91.032123

[16] F. Brandão, M. Horodecki, N. Ng, J. Oppenheim, and S. Wehner, ``The second laws of quantum thermodynamics,'' Proceedings of the National Academy of Sciences 112, 3275–3279 (2015).
https://doi.org/10.1073/pnas.1411728112

[17] R. García-Patrón, C. Navarrete-Benlloch, S. Lloyd, J. H. Shapiro, and N. J. Cerf, ``Majorization Theory Approach to the Gaussian Channel Minimum Entropy Conjecture,'' Physical Review Letters 108, 110505 (2012).
https://doi.org/10.1103/PhysRevLett.108.110505

[18] C. N. Gagatsos, O. Oreshkov, and N. J. Cerf, ``Majorization relations and entanglement generation in a beam splitter,'' Physical Review A 87, 042307 (2013).
https://doi.org/10.1103/PhysRevA.87.042307

[19] G. De Palma, D. Trevisan, and V. Giovannetti, ``Passive States Optimize the Output of Bosonic Gaussian Quantum Channels,'' IEEE Transactions on Information Theory 62, 2895–2906 (2016).
https://doi.org/10.1109/TIT.2016.2547426

[20] M. G. Jabbour, R. García-Patrón, and N. J. Cerf, ``Majorization preservation of Gaussian bosonic channels,'' New Journal of Physics 18, 073047 (2016).
https://doi.org/10.1088/1367-2630/18/7/073047

[21] M. G. Jabbour and N. J. Cerf, ``Fock majorization in bosonic quantum channels with a passive environment,'' Journal of Physics A: Mathematical and Theoretical 52, 105302 (2019).
https://doi.org/10.1088/1751-8121/aaf0d2

[22] U. Leonhardt, ``Essential quantum optics: from quantum measurements to black holes,''. Cambridge University Press, 2010.
https://doi.org/10.1017/CBO9780511806117

[23] A. Hertz, M. G. Jabbour, and N. J. Cerf, ``Entropy-power uncertainty relations: towards a tight inequality for all Gaussian pure states,'' Journal of Physics A: Mathematical and Theoretical 50, 385301 (2017).
https://doi.org/10.1088/1751-8121/aa852f

[24] A. Hertz and N. J. Cerf, ``Continuous-variable entropic uncertainty relations,'' Journal of Physics A: Mathematical and Theoretical 52, 173001 (2019).
https://doi.org/10.1088/1751-8121/ab03f3

[25] C. Weedbrook, S. Pirandola, R. García-Patrón, N. J. Cerf, T. C. Ralph, J. H. Shapiro, and S. Lloyd, ``Gaussian quantum information,'' Review of Modern Physics 84, 621–669 (2012).
https://doi.org/10.1103/RevModPhys.84.621

[26] Z. Van Herstraeten and N. J. Cerf, ``Quantum Wigner entropy,'' Physical Review A 104, 042211 (2021).
https://doi.org/10.1103/PhysRevA.104.042211

[27] F. J. Narcowich, ``Distributions of $hbar$-positive type and applications,'' Journal of mathematical physics 30, 2565–2573 (1989).
https://doi.org/10.1063/1.528537

[28] T. Bröcker and R. Werner, ``Mixed states with positive Wigner functions,'' Journal of mathematical physics 36, 62–75 (1995).
https://doi.org/10.1063/1.531326

[29] RL Hudson, ``ความหนาแน่นเสมือนความน่าจะเป็นของ Wigner ไม่เป็นลบเมื่อใด,'' รายงานเกี่ยวกับฟิสิกส์คณิตศาสตร์ 6, 249–252 (1974)
https://doi.org/10.1016/0034-4877(74)90007-X

[30] F. Soto และ P. Claverie, ``ฟังก์ชัน Wigner ของระบบหลายมิติจะไม่เป็นลบเมื่อใด,'' Journal of Mathematical Physics 24, 97–100 (1983)
https://doi.org/10.1063/1.525607

[31] F. J. Narcowich and R. O’Connell, ``Necessary and sufficient conditions for a phase-space function to be a Wigner distribution,'' Physical Review A 34, 1 (1986).
https://doi.org/10.1103/PhysRevA.34.1

[32] A. Mandilara, E. Karpov, and N. J. Cerf, ``Extending Hudson's theorem to mixed quantum states,'' Physical Review A 79, 062302 (2009).
https://doi.org/10.1103/PhysRevA.79.062302

[33] A. Mandilara, E. Karpov, and N. Cerf, ``Gaussianity bounds for quantum mixed states with a positive Wigner function,'' in Journal of Physics: Conference Series, vol. 254, p. 012011, IOP Publishing. 2010.
https://doi.org/10.1088/1742-6596/254/1/012011

[34] L. Wang and M. Madiman, ``Beyond the Entropy Power Inequality, via Rearrangements,'' IEEE Transactions on Information Theory 60, 5116–5137 (2014).
https://doi.org/10.1109/TIT.2014.2338852

[35] G. H. Hardy, J. E. Littlewood, and G. Pólya, ``Some simple inequalities satisfied by convex functions,'' Messenger of Mathematics 58, 145–152 (1929).

[36] H. Joe, ``An ordering of dependence for distribution of k-tuples, with applications to lotto games,'' Canadian Journal of Statistics 15, 227–238 (1987).
https://doi.org/10.2307/3314913

[37] I. Schur, ``Uber eine Klasse von Mittelbildungen mit Anwendungen die Determinanten,'' Sitzungsberichte der Berliner Mathematischen Gesellschaft 22, 416–427 (1923).

[38] A. W. Roberts and D. E. Varberg, ``Convex functions,''. Academic Press New York, 1973.
https://doi.org/10.1016/B978-0-444-89597-4.50013-5

[39] A. Rényi, ``On measures of entropy and information,'' in Proceedings of the Fourth Berkeley Symposium on Mathematical Statistics and Probability, Volume 1: Contributions to the Theory of Statistics, vol. 4, pp. 547–562, University of California Press. 1961.

[40] Y. He, A. B. Hamza, and H. Krim, ``A generalized divergence measure for robust image registration,'' IEEE Transactions on Signal Processing 51, 1211–1220 (2003).
https://doi.org/10.1109/TSP.2003.810305

[41] J. V. Ryff, ``Orbits of $L^1$-functions under doubly stochastic transformations,'' Transactions of the American Mathematical Society 117, 92–100 (1965).
https://doi.org/10.2307/1994198

[42] F. Bahrami, S. M. Manjegani, and S. Moein, ``Semi-doubly Stochastic Operators and Majorization of Integrable Functions,'' Bulletin of the Malaysian Mathematical Sciences Society 44, 693–703 (2021).
https://doi.org/10.1007/s40840-020-00971-2

[43] S. M. Manjegani and S. Moein, ``Majorization and semidoubly stochastic operators on $ L^{1}(X)$,'' Journal of Inequalities and Applications 2023, 1–20 (2023).
https://doi.org/10.1186/s13660-023-02935-z

[44] I. Białynicki-Birula and J. Mycielski, ``Uncertainty relations for information entropy in wave mechanics,'' Communications in Mathematical Physics 44, 129–132 (1975).
https://doi.org/10.1007/BF01608825

[45] A. Wehrl, ``General properties of entropy,'' Reviews of Modern Physics 50, 221 (1978).
https://doi.org/10.1103/RevModPhys.50.221

[46] E. H. Lieb, ``Proof of an entropy conjecture of Wehrl,'' in Inequalities, pp. 359–365. Springer, 2002.
https://doi.org/10.1007/978-3-642-55925-9_30

[47] E. H. Lieb and J. P. Solovej, ``Proof of an entropy conjecture for Bloch coherent spin states and its generalizations,'' Acta Mathematica 212, 379 (2014).
https://doi.org/10.1007/s11511-014-0113-6

[48] J. R. Johansson, P. D. Nation, and F. Nori, ``QuTiP: An open-source Python framework for the dynamics of open quantum systems,'' Computer Physics Communications 183, 1760–1772 (2012).
https://doi.org/10.1016/j.cpc.2012.02.021

[49] K. Życzkowski, P. Horodecki, A. Sanpera, and M. Lewenstein, ``Volume of the set of separable states,'' Physical Review A 58, 883 (1998).
https://doi.org/10.1103/PhysRevA.58.883

อ้างโดย

[1] Nuno Costa Dias and João Nuno Prata, "On a recent conjecture by Z. Van Herstraeten and N.J. Cerf for the quantum Wigner entropy", arXiv: 2303.10531, (2023).

[2] Zacharie Van Herstraeten and Nicolas J. Cerf, "Quantum Wigner entropy", การตรวจร่างกาย A 104 4, 042211 (2021).

[3] Martin Gärttner, Tobias Haas, and Johannes Noll, "Detecting continuous variable entanglement in phase space with the $Q$-distribution", arXiv: 2211.17165, (2022).

การอ้างอิงข้างต้นมาจาก are อบต./นาซ่าโฆษณา (ปรับปรุงล่าสุดสำเร็จ 2023-05-24 23:55:18 น.) รายการอาจไม่สมบูรณ์เนื่องจากผู้จัดพิมพ์บางรายไม่ได้ให้ข้อมูลอ้างอิงที่เหมาะสมและครบถ้วน

On บริการอ้างอิงของ Crossref ไม่พบข้อมูลอ้างอิงงาน (ความพยายามครั้งสุดท้าย 2023-05-24 23:55:17)

บทความนี้เผยแพร่ใน Quantum ภายใต้ the ครีเอทีฟคอมมอนส์แบบแสดงที่มา 4.0 สากล (CC BY 4.0) ใบอนุญาต ลิขสิทธิ์ยังคงอยู่กับผู้ถือลิขสิทธิ์ดั้งเดิม เช่น ผู้เขียนหรือสถาบันของพวกเขา

เนื้อหาที่ขับเคลื่อนด้วย SEO และการเผยแพร่ประชาสัมพันธ์ รับการขยายวันนี้
เพลโตไอสตรีม. ข้อมูลอัจฉริยะ Web3 ขยายความรู้ เข้าถึงได้ที่นี่.
การสร้างอนาคตโดย Adryenn Ashley เข้าถึงได้ที่นี่.
ซื้อและขายหุ้นในบริษัท PRE-IPO ด้วย PREIPO® เข้าถึงได้ที่นี่.
ที่มา: https://quantum-journal.org/papers/q-2023-05-24-1021/

ประทับเวลา: May 24, 2023

ประทับเวลา: มิถุนายน 5, 2023

วิชาเอกต่อเนื่องในพื้นที่เฟสควอนตัม

เผยแพร่ซ้ำโดยเพลโต

นามธรรม

สรุปยอดนิยม

► ข้อมูล BibTeX

► ข้อมูลอ้างอิง

อ้างโดย

เพิ่มเติมจาก วารสารควอนตัม

การเปลี่ยนแปลงการแยกส่วนตัวถังแบบไดนามิกของก๊าซ Tonks ในศักยภาพการขับขี่แบบกึ่งคาบ

ประตูลอจิกควอนตัมสำหรับอิเล็กตรอนอิสระ

อัลกอริธึมควอนตัมจากทฤษฎีบทความผันผวน: การเตรียมสภาวะความร้อน

การออกแบบช่องควอนตัมที่เกิดจาก Diagonal Gates

สูตรหลายผลิตภัณฑ์ที่มีเงื่อนไขอย่างดีสำหรับการจำลองแบบแฮมิลตันที่เป็นมิตรต่อฮาร์ดแวร์

การรวบรวมวงจรควอนตัมและการคำนวณแบบไฮบริดโดยใช้การคำนวณแบบเพาลี

การตรวจสอบความพัวพันของควอนตัมจากประชากรในระดับแม่เหล็ก: นอกเหนือไปจากความไม่เท่าเทียมกันของสปิน

การวัดทอพอโลยีฟังก์ชันคลื่นที่มีประสิทธิภาพบนคอมพิวเตอร์ควอนตัม NISQ

การผลิตเอนโทรปีพัวพันในเครือข่ายประสาทควอนตัม

กฎการรบกวนการวัดและการอนุรักษ์ในกลศาสตร์ควอนตัม

เกี่ยวกับเรา

การค้นหาแนวตั้ง & Ai

ระบบปฏิบัติการ

การติดต่อ

ลงชื่อเข้าใช้