1Institute for Quantum Optics and Quantum Information Vienna, Boltzmanngasse 3 1090 Vienna, Austria
2Institute for Computational and Experimental Research in Mathematics, 121 South Main Street Providence RI 02903, USA
3Max Planck Institute for Mathematics in the Sciences Leipzig, Inselstrasse 22 04103 Leipzig, Germany
4Department of Mathematics, University of California, Berkeley, 970 Evans Hall #3840 Berkeley CA 94720-3840, USA
5Insitute für Theoretische Physik, Leibniz Universität Hannover, Appelstrasse 2 30167 Hannover, Germany
Find this paper interesting or want to discuss? Scite or leave a comment on SciRate.
Abstract
In the minimal scenario of quantum correlations, two parties can choose from two observables with two possible outcomes each. Probabilities are specified by four marginals and four correlations. The resulting four-dimensional convex body of correlations, denoted $mathcal{Q}$, is fundamental for quantum information theory. We review and systematize what is known about $mathcal{Q}$, and add many details, visualizations, and complete proofs. In particular, we provide a detailed description of the boundary, which consists of three-dimensional faces isomorphic to elliptopes and sextic algebraic manifolds of exposed extreme points. These patches are separated by cubic surfaces of non-exposed extreme points. We provide a trigonometric parametrization of all extreme points, along with their exposing Tsirelson inequalities and quantum models. All non-classical extreme points (exposed or not) are self-testing, i.e., realized by an essentially unique quantum model.
Two principles, which are specific to the minimal scenario, allow a quick and complete overview: The first is the pushout transformation, i.e., the application of the sine function to each coordinate. This transforms the classical correlation polytope exactly into the correlation body $mathcal{Q}$, also identifying the boundary structures. The second principle, self-duality, is an isomorphism between $mathcal{Q}$ and its polar dual, i.e., the set of affine inequalities satisfied by all quantum correlations (“Tsirelson inequalities''). The same isomorphism links the polytope of classical correlations contained in $mathcal{Q}$ to the polytope of no-signalling correlations, which contains $mathcal{Q}$.
We also discuss the sets of correlations achieved with fixed Hilbert space dimension, fixed state or fixed observables, and establish a new non-linear inequality for $mathcal{Q}$ involving the determinant of the correlation matrix.
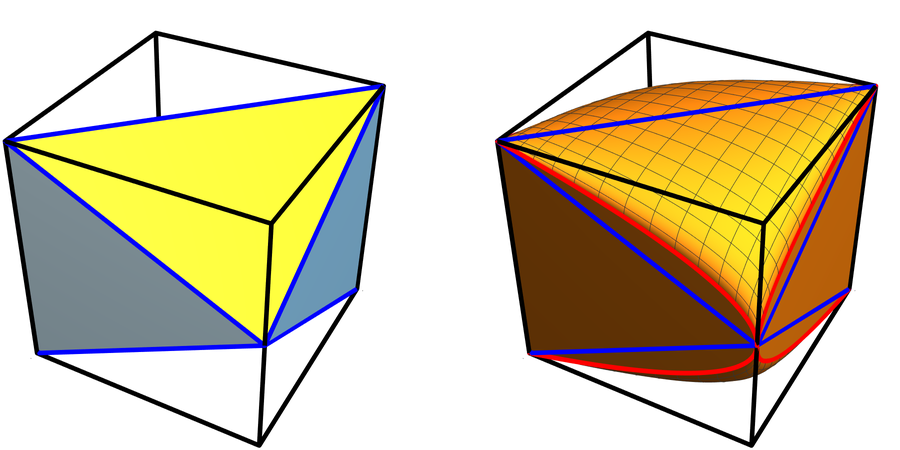
Featured image: The relationship of three correlation sets: classical and no-signalling polytopes (left) and quantum set (right, orange).
Popular summary
► BibTeX data
► References
[1] Alain Aspect, Philippe Grangier, and Gérard Roger. ``Experimental realization of Einstein-Podolsky-Rosen-Bohm Gedankenexperiment: A new violation of Bell's inequalities''. Phys. Rev. Lett. 49, 91–94 (1982).
https://doi.org/10.1103/PhysRevLett.49.91
[2] B. Hensen, R. Hanson, et al. ``Loophole-free Bell inequality violation using electron spins separated by 1.3 kilometres''. Nature 526, 682 EP – (2015). arXiv:1508.05949.
https://doi.org/10.1038/nature15759
arXiv:1508.05949
[3] N. Sangouard, J.-D. Bancal, N. Gisin, W. Rosenfeld, P. Sekatski, M. Weber, and H. Weinfurter. ``Loophole-free Bell test with one atom and less than one photon on average''. Phys. Rev. A 84, 052122 (2011). arXiv:1108.1027.
https://doi.org/10.1103/PhysRevA.84.052122
arXiv:1108.1027
[4] J. S. Bell. ``On the Einstein Podolsky Rosen paradox''. Physics 1, 195–200 (1964).
https://doi.org/10.1103/PhysicsPhysiqueFizika.1.195
[5] John F. Clauser, Michael A. Horne, Abner Shimony, and Richard A. Holt. ``Proposed experiment to test local hidden-variable theories''. Phys. Rev. Lett. 23, 880–884 (1969).
https://doi.org/10.1103/PhysRevLett.23.880
[6] R. F. Werner et al. ``Open quantum problems''. url: https://oqp.iqoqi.oeaw.ac.at/.
https://oqp.iqoqi.oeaw.ac.at/
[7] Boris S. Tsirelson. ``Quantum analogues of the Bell inequalities. the case of two spatially separated domains''. J. Soviet Math. 36, 557–570 (1987).
https://doi.org/10.1007/BF01663472
[8] R. F. Werner and M. M. Wolf. ``All multipartite Bell-correlation inequalities for two dichotomic observables per site''. Phys. Rev. A 64, 032112 (2001). arXiv:quant-ph/0102024.
https://doi.org/10.1103/PhysRevA.64.032112
arXiv:quant-ph/0102024
[9] William Slofstra. ``The set of quantum correlations is not closed''. Forum of Mathematics, Pi 7, e1 (2019). arXiv:1703.08618.
https://doi.org/10.1017/fmp.2018.3
arXiv:1703.08618
[10] Volkher B. Scholz and R. F. Werner. ``Tsirelson's problem'' (2008). arXiv:0812.4305.
arXiv:0812.4305
[11] Boris S Tsirelson. ``Some results and problems on quantum Bell-type inequalities''. Hadronic Journal Supplement 8, 329–345 (1993). url: https://www.tau.ac.il/ tsirel/download/hadron.html.
https://www.tau.ac.il/~tsirel/download/hadron.html
[12] Miguel Navascues, Stefano Pironio, and Antonio Acín. ``A convergent hierarchy of semidefinite programs characterizing the set of quantum correlations''. New J. Phys. 10, 073013 (2008).
https://doi.org/10.1088/1367-2630/10/7/073013
[13] M. Junge, M. Navascues, C. Palazuelos, D. Perez-Garcia, V. B. Scholz, and R. F. Werner. ``Connes' embedding problem and Tsirelson's problem''. J. Math. Phys. 52, 012102 (2011). arXiv:1008.1142.
https://doi.org/10.1063/1.3514538
arXiv:1008.1142
[14] Tobias Fritz. ``Tsirelson's problem and Kirchberg's conjecture''. Rev. Math. Phys. 24, 1250012 (2012). arXiv:1008.1168.
https://doi.org/10.1142/S0129055X12500122
arXiv:1008.1168
[15] Zhengfeng Ji, Anand Natarajan, Thomas Vidick, John Wright, and Henry Yuen. ``MIP*=RE'' (2020). arXiv:2001.04383.
arXiv:2001.04383
[16] Günther M. Ziegler. ``Lectures on polytopes''. Springer. Berlin (1995).
https://doi.org/10.1007/978-1-4613-8431-1
[17] Mateusz Michałek and Bernd Sturmfels. ``Invitation to nonlinear algebra''. Volume 211 of Graduate Studies in Mathematics. AMS. (2021).
https://doi.org/10.1007/s00591-022-00324-z
[18] Grigoriy Blekherman, Pablo Parrilo, and Rekha Thomas. ``Semidefinite optimization and convex algebraic geometry''. MOS-SIAM Series on Optimization 13. SIAM. Philadelphia (2012).
https://doi.org/10.1137/1.9781611972290
[19] Bernd Sturmfels and Caroline Uhler. ``Multivariate Gaussians, semidefinite matrix completion, and convex algebraic geometry''. Ann. Inst. Statist. Math. 62, 603–638 (2010). arXiv:0906.3529.
https://doi.org/10.1007/s10463-010-0295-4
arXiv:0906.3529
[20] Claus Scheiderer. ``Spectrahedral shadows''. SIAM J. Appl. Algebra Geometry 2, 26–44 (2018). arXiv:1612.07048.
https://doi.org/10.1137/17M1118981
arXiv:1612.07048
[21] B. S. Cirel'son. ``Quantum generalizations of Bell's inequality''. Lett. Math. Phys. 4, 93–100 (1980).
https://doi.org/10.1007/BF00417500
[22] Jukka Kiukas and Reinhard F. Werner. ``Maximal violation of Bell inequalities by position measurements''. J. Math. Phys. 51, 072105 (2010). arXiv:0912.3740.
https://doi.org/10.1063/1.3447736
arXiv:0912.3740
[23] Lawrence J. Landau. ``Empirical two-point correlation functions''. Found. Phys. 18, 449–460 (1988).
https://doi.org/10.1007/BF00732549
[24] L Masanes. ``Necessary and sufficient condition for quantum-generated correlations'' (2003) arXiv:quant-ph/0309137.
arXiv:quant-ph/0309137
[25] Yukun Wang, Xingyao Wu, and Valerio Scarani. ``All the self-testings of the singlet for two binary measurements''. New J. Phys. 18, 025021 (2016). arXiv:1511.04886.
https://doi.org/10.1088/1367-2630/18/2/025021
arXiv:1511.04886
[26] Andrew C Doherty, Yeong-Cherng Liang, Ben Toner, and Stephanie Wehner. ``The quantum moment problem and bounds on entangled multi-prover games''. In 23rd Annual IEEE Conference on Computational Complexity. Pages 199–210. IEEE (2008). arXiv:0803.4373.
https://doi.org/10.1109/CCC.2008.26
arXiv:0803.4373
[27] Tobias Fritz. ``Polyhedral duality in Bell scenarios with two binary observables''. J. Math. Phys. 53, 072202 (2012). arXiv:1202.0141.
https://doi.org/10.1063/1.4734586
arXiv:1202.0141
[28] Dominic Mayers and Andrew Yao. ``Self testing quantum apparatus''. Quantum Info. Comput. 4, 273–286 (2004). arXiv:quant-ph/0307205.
https://doi.org/10.26421/QIC4.4-3
arXiv:quant-ph/0307205
[29] Stephen J. Summers and Reinhard F. Werner. ``Maximal violation of Bell's inequalities is generic in quantum field theory''. Commun. Math. Phys. 110, 247–259 (1987).
https://doi.org/10.1007/BF01207366
[30] L Masanes. ``Extremal quantum correlations for n parties with two dichotomic observables per site'' (2005) arXiv:quant-ph/0512100.
arXiv:quant-ph/0512100
[31] Le Phuc Thinh, Antonios Varvitsiotis, and Yu Cai. ``Geometric structure of quantum correlators via semidefinite programming''. Phys. Rev. A 99, 052108 (2019). arXiv:1809.10886.
https://doi.org/10.1103/PhysRevA.99.052108
arXiv:1809.10886
[32] Nicolas Brunner, Daniel Cavalcanti, Stefano Pironio, Valerio Scarani, and Stephanie Wehner. ``Bell nonlocality''. Rev. Mod. Phys. 86, 419–478 (2014). arXiv:1303.2849.
https://doi.org/10.1103/RevModPhys.86.419
arXiv:1303.2849
[33] Koon Tong Goh, Jędrzej Kaniewski, Elie Wolfe, Tamás Vértesi, Xingyao Wu, Yu Cai, Yeong-Cherng Liang, and Valerio Scarani. ``Geometry of the set of quantum correlations''. Phys. Rev. A 97, 022104 (2018). arXiv:1710.05892.
https://doi.org/10.1103/PhysRevA.97.022104
arXiv:1710.05892
[34] Ivan Šupić and Joseph Bowles. ``Self-testing of quantum systems: a review''. Quantum 4, 337 (2020). arXiv:1904.10042.
https://doi.org/10.22331/q-2020-09-30-337
arXiv:1904.10042
[35] Rene Schwonnek, Koon Tong Goh, Ignatius W. Primaatmaja, Ernest Y. Z. Tan, Ramona Wolf, Valerio Scarani, and Charles C. W. Lim. ``Device-independent quantum key distribution with random key basis''. Nat. Commun. 12, 2880 (2020). arXiv:2005.02691.
https://doi.org/10.1038/s41467-021-23147-3
arXiv:2005.02691
[36] Ernest Y. Z. Tan, René Schwonnek, Koon Tong Goh, Ignatius William Primaatmaja, and Charles C. W. Lim. ``Computing secure key rates for quantum key distribution with untrusted devices''. npj Quantum Inf. 7, 158 (2021). arXiv:1908.11372.
https://doi.org/10.1038/s41534-021-00494-z
arXiv:1908.11372
[37] K. G. H. Vollbrecht and R. F. Werner. ``Entanglement measures under symmetry''. Phys. Rev. A 64, 062307 (2001). arXiv:quant-ph/0010095.
https://doi.org/10.1103/PhysRevA.64.062307
arXiv:quant-ph/0010095
[38] Peter Bierhorst. ``Geometric decompositions of Bell polytopes with practical applications''. J. Phys. A 49, 215301 (2016). arXiv:1511.04127.
https://doi.org/10.1088/1751-8113/49/21/215301
arXiv:1511.04127
[39] Monique Laurent. ``The real positive semidefinite completion problem for series-parallel graphs''. Linear Algebra and its Applications 252, 347–366 (1997).
https://doi.org/10.1016/0024-3795(95)00741-5
[40] Vaughan F. R. Jones and J. H. Przytycki. ``Lissajous knots and billiard knots''. Banach Cent. Pub. 42, 145–163 (1998).
https://doi.org/10.4064/-42-1-145-163
[41] Kaie Kubjas, Pablo A Parrilo, and Bernd Sturmfels. ``How to flatten a soccer ball''. In Aldo Conca, Joseph Gubeladze, and Tim Römer, editors, Homological and Computational Methods in Commutative Algebra. Volume 20 of INdAM Ser., pages 141–162. Springer (2017).
https://doi.org/10.1007/978-3-319-61943-9_9
[42] Kathleen S. Gibbons, Matthew J. Hoffman, and William K. Wootters. ``Discrete phase space based on finite fields''. Phys. Rev. A 70, 062101 (2004). arXiv:quant-ph/0401155.
https://doi.org/10.1103/physreva.70.062101
arXiv:quant-ph/0401155
[43] Reinhard F. Werner. ``Uncertainty relations for general phase spaces''. Frontiers of Physics 11, 1–10 (2016). arXiv:arxiv:1601.03843.
https://doi.org/10.1007/s11467-016-0558-5
arXiv:1601.03843
[44] Amritanshu Prasad, Ilya Shapiro, and M.K. Vemuri. ``Locally compact abelian groups with symplectic self-duality''. Adv. Math. 225, 2429–2454 (2010). arXiv:0906.4397.
https://doi.org/10.1016/j.aim.2010.04.023
arXiv:0906.4397
[45] Daniel Ciripoi, Nidhi Kaihnsa, Andreas Löhne, and Bernd Sturmfels. ``Computing convex hulls of trajectories''. Rev. Un. Mat. Argentina 60, 637–662 (2019). arXiv:1810.03547.
https://doi.org/10.33044/revuma.v60n2a22
arXiv:1810.03547
[46] Daniel Plaumann, Rainer Sinn, and Jannik Lennart Wesner. ``Families of faces and the normal cycle of a convex semi-algebraic set''. Beitr. Algebra Geom. (2022). arXiv:2104.13306.
https://doi.org/10.1007/s13366-022-00657-9
arXiv:2104.13306
[47] Daniel R. Grayson and Michael E. Stillman. ``Macaulay2, a software system for research in algebraic geometry''. Available at http://www.math.uiuc.edu/Macaulay2/.
http://www.math.uiuc.edu/Macaulay2/
[48] John Ottem, Kristian Ranestad, Bernd Sturmfels, and Cynthia Vinzant. ``Quartic spectrahedra''. Mathematical Programming, Ser. B 151, 585–612 (2015). arXiv:1311.3675.
https://doi.org/10.1007/s10107-014-0844-3
arXiv:1311.3675
[49] Adán Cabello. ``How much larger quantum correlations are than classical ones''. Phys. Rev. A 72, 012113 (2005). arXiv:quant-ph/0409192.
https://doi.org/10.1103/PhysRevA.72.012113
arXiv:quant-ph/0409192
[50] C. E. González-Guillén, C. H. Jiménez, C. Palazuelos, and I. Villanueva. ``Sampling quantum nonlocal correlations with high probability''. Commun. Math. Phys. 344, 141–154 (2016). arXiv:1412.4010.
https://doi.org/10.1007/s00220-016-2625-8
arXiv:1412.4010
[51] C. R. Johnson and G. Nævdal. ``The probability that a (partial) matrix is positive semidefinite''. In I. Gohberg, R. Mennicken, and C. Tretter, editors, Recent Progress in Operator Theory. Pages 171–182. Basel (1998). Birkhäuser Basel.
https://doi.org/10.1007/978-3-0348-8793-9_10
[52] H. H Schaefer and M. P Wolff. ``Topological vector spaces''. Springer. (1999).
https://doi.org/10.1007/978-1-4612-1468-7
[53] Wojciech Tadej and Karol Z̀yczkowski. ``A concise guide to complex Hadamard matrices''. Open Systems & Information Dynamics 13, 133–177 (2006). arXiv:quant-ph/0512154.
https://doi.org/10.1007/s11080-006-8220-2
arXiv:quant-ph/0512154
[54] H. Barnum, C.P. Gaebler, and A. Wilce. ``Ensemble steering, weak self-duality, and the structure of probabilistic theories''. Found. Phys 43, 1411–1427 (2013). arXiv:0912.5532.
https://doi.org/10.1007/s10701-013-9752-2
arXiv:0912.5532
[55] Nikos Yannakakis. ``Stampacchia's property, self-duality and orthogonality relations''. Set-Valued and Variational Analysis 19, 555–567 (2011). arXiv:1008.4958.
https://doi.org/10.1007/s11228-011-0175-y
arXiv:1008.4958
[56] Jacek Bochnak, Michel Coste, and Marie-Françoise Roy. ``Real algebraic geometry''. Volume 36 of A Series of Modern Surveys in Mathematics. Springer Berlin, Heidelberg. (2013).
https://doi.org/10.1007/978-3-662-03718-8
[57] Joseph H. G. Fu. ``Algebraic integral geometry''. Pages 47–112. Springer Basel. Basel (2014). arXiv:1103.6256.
https://doi.org/10.1007/978-3-0348-0874-3_2
arXiv:1103.6256
[58] Herbert Federer. ``Curvature measures''. Trans. Amer. Math. Soc. 93, 418–491 (1959).
https://doi.org/10.2307/1993504
[59] Peter Wintgen. ``Normal cycle and integral curvature for polyhedra in Riemannian manifolds''. In Gy. Soos and J. Szenthe, editors, Differential Geometry. Volume 21. North-Holland, Amsterdam (1982).
[60] Martina Zähle. ``Integral and current representation of Federer's curvature measures''. Arch. Math. 46, 557–567 (1986).
https://doi.org/10.1007/BF01195026
[61] David Cohen-Steiner and Jean-Marie Morvan. ``Restricted Delaunay triangulations and normal cycle''. In SCG '03: Proceedings of the nineteenth annual symposium on Computational geometry. Pages 312–321. (2003).
https://doi.org/10.1145/777792.777839
[62] Pierre Roussillon and Joan Alexis Glaunès. ``Surface matching using normal cycles''. In Frank Nielsen and Frédéric Barbaresco, editors, Geometric Science of Information. Pages 73–80. Cham (2017). Springer International Publishing.
https://doi.org/10.1007/978-3-319-68445-1_9
[63] Kehua Su, Na Lei, Wei Chen, Li Cui, Hang Si, Shikui Chen, and Xianfeng Gu. ``Curvature adaptive surface remeshing by sampling normal cycle''. Computer-Aided Design 111, 1–12 (2019).
https://doi.org/10.1016/j.cad.2019.01.004
[64] David A. Cox, John Little, and Donal O'Shea. ``Ideals, varieties, and algorithms''. Undergraduate Texts in Mathematics. Springer Cham. (2015). Fourth edition.
https://doi.org/10.1007/978-3-319-16721-3
[65] Guido A. Raggio. ``A remark on Bell's inequality and decomposable normal states''. Lett. Math. Phys. 15, 27–29 (1988).
https://doi.org/10.1007/BF00416568
[66] Marc-Olivier Renou, David Trillo, Mirjam Weilenmann, Thinh P. Le, Armin Tavakoli, Nicolas Gisin, Antonio Acín, and Miguel Navascués. ``Quantum theory based on real numbers can be experimentally falsified''. Nature 600, 625–629 (2021). arXiv:2101.10873.
https://doi.org/10.1038/s41586-021-04160-4
arXiv:2101.10873
[67] Andrea Coladangelo, Koon Tong Goh, and Valerio Scarani. ``All pure bipartite entangled states can be self-tested''. Nature Commun. 8, 15485 (2017). arXiv:1611.08062.
https://doi.org/10.1038/ncomms15485
arXiv:1611.08062
[68] Charles H. Bennett and Gilles Brassard. ``Quantum cryptography: Public key distribution and coin tossing''. Theoret. Comp. Sci. 560, 7–11 (2014). arXiv:2003.06557.
https://doi.org/10.1016/j.tcs.2014.05.025
arXiv:2003.06557
[69] T. Franz, F. Furrer, and R. F. Werner. ``Extremal quantum correlations and cryptographic security''. Phys. Rev. Lett. 106, 250502 (2011). arXiv:1010.1131.
https://doi.org/10.1103/PhysRevLett.106.250502
arXiv:1010.1131
[70] Jędrzej Kaniewski. ``Weak form of self-testing''. Phys. Rev. Research 2, 033420 (2020). arXiv:1910.00706.
https://doi.org/10.1103/PhysRevResearch.2.033420
arXiv:1910.00706
[71] C. H. Bennett, G. Brassard, C. Crepeau, and U. M. Maurer. ``Generalized privacy amplification''. IEEE Transactions on Information Theory 41, 1915–1923 (1995).
https://doi.org/10.1109/18.476316
[72] Pavel Sekatski, Jean-Daniel Bancal, Xavier Valcarce, Ernest Y.-Z. Tan, Renato Renner, and Nicolas Sangouard. ``Device-independent quantum key distribution from generalized CHSH inequalities''. Quantum 5, 444 (2021). arXiv:2009.01784.
https://doi.org/10.22331/q-2021-04-26-444
arXiv:2009.01784
[73] Ernest Y.-Z. Tan, Pavel Sekatski, Jean-Daniel Bancal, René Schwonnek, Renato Renner, Nicolas Sangouard, and Charles C.-W. Lim. ``Improved DIQKD protocols with finite-size analysis''. Quantum 6, 880 (2022). arXiv:2012.08714.
https://doi.org/10.22331/q-2022-12-22-880
arXiv:2012.08714
[74] Marissa Giustina et al. ``Significant-loophole-free test of Bell's theorem with entangled photons''. Phys. Rev. Lett. 115, 250401 (2015). arXiv:1511.03190.
https://doi.org/10.1103/PhysRevLett.115.250401
arXiv:1511.03190
[75] Lynden K. Shalm et al. ``Strong loophole-free test of local realism''. Phys. Rev. Lett. 115, 250402 (2015). arXiv:1511.03189.
https://doi.org/10.1103/PhysRevLett.115.250402
arXiv:1511.03189
[76] D. P Nadlinger, J.-D. Bancal, and et al. ``Experimental quantum key distribution certified by Bell's theorem''. Nature 607, 682–686 (2022). arXiv:2109.14600.
https://doi.org/10.1038/s41586-022-04941-5
arXiv:2109.14600
[77] Wei Zhang, Harald Weinfurter, et al. ``A device-independent quantum key distribution system for distant users''. Nature 607, 687–691 (2022). arXiv:2110.00575.
https://doi.org/10.1038/s41586-022-04891-y
arXiv:2110.00575
[78] Feihu Xu, Yu-Zhe Zhang, Qiang Zhang, and Jian-Wei Pan. ``Device-independent quantum key distribution with random postselection''. Phys. Rev. Lett. 128, 110506 (2022). arXiv:2110.02701.
https://doi.org/10.1103/PhysRevLett.128.110506
arXiv:2110.02701
[79] Wikipedia authors. ``Quantum key distribution''. url: https://en.wikipedia.org/wiki/Quantum_key_distribution. (accessed: 25-October-2021).
https://en.wikipedia.org/wiki/Quantum_key_distribution
[80] Armin Tavakoli, Máté Farkas, Denis Rosset, Jean-Daniel Bancal, and Jedrzej Kaniewski. ``Mutually unbiased bases and symmetric informationally complete measurements in Bell experiments''. Science Advances 7, eabc3847 (2021). arXiv:1912.03225.
https://doi.org/10.1126/sciadv.abc3847
arXiv:1912.03225
[81] Stephen J. Summers and Reinhard F. Werner. ``Maximal violation of Bell's inequalities for algebras of observables in tangent spacetime regions''. Ann. Inst. H. Poincaré. 49, 215–243 (1988).
[82] N. David Mermin. ``Is the moon there when nobody looks? Reality and the quantum theory''. Physics Today 38, 38–47 (1985).
https://doi.org/10.1063/1.880968
[83] Michael Janas, Michael E. Cuffaro, and Michel Janssen. ``Putting probabilities first. How Hilbert space generates and constrains them'' (2019) arXiv:1910.10688.
arXiv:1910.10688
[84] Nicolas Brunner, Stefano Pironio, Antonio Acín, Nicolas Gisin, André Allan Méthot, and Valerio Scarani. ``Testing the dimension of Hilbert spaces''. Phys. Rev. Lett. 100, 210503 (2008). arXiv:0802.0760.
https://doi.org/10.1103/PhysRevLett.100.210503
arXiv:0802.0760
[85] Yu Cai, Jean-Daniel Bancal, Jacquiline Romero, and Valerio Scarani. ``A new device-independent dimension witness and its experimental implementation''. J. Phys. A 49, 305301 (2016). arXiv:1606.01602.
https://doi.org/10.1088/1751-8113/49/30/305301
arXiv:1606.01602
[86] Wan Cong, Yu Cai, Jean-Daniel Bancal, and Valerio Scarani. ``Witnessing irreducible dimension''. Phys. Rev. Lett. 119, 080401 (2017). arXiv:1611.01258.
https://doi.org/10.1103/PhysRevLett.119.080401
arXiv:1611.01258
[87] R. Horodecki, P. Horodecki, and M. Horodecki. ``Violating Bell inequality by mixed spin-1/2 states: necessary and sufficient condition''. Phys. Lett. A 200, 340–344 (1995).
https://doi.org/10.1016/0375-9601(95)00214-N
[88] N. Gisin. ``Bell's inequality holds for all non-product states''. Physics Letters A 154, 201–202 (1991).
https://doi.org/10.1016/0375-9601(91)90805-I
[89] R. Grone, C.R. Johnson, E.M. Sá, and H. Wolkowicz. ``Positive definite completions of partial Hermitian matrices''. Lin. Alg. Appl. 58, 109–124 (1984).
https://doi.org/10.1016/0024-3795(84)90207-6
[90] Alexander Barvinok. ``A course in convexity''. Graduate Studies in Mathematics 54. AMS. Providence (2002).
https://doi.org/10.1090/gsm/054
[91] J. Dixmier. ``C*-algebras''. North-Holland mathematical library. North-Holland. (1982).
[92] M. Reed and B. Simon. ``Methods of modern mathematical physics IV: Analysis of operators''. Elsevier Science. (1978).
[93] Iain Raeburn and Allan M. Sinclair. ``The C*-algebra generated by two projections.''. Math. Scand. 65, 278–290 (1989).
https://doi.org/10.7146/math.scand.a-12283
[94] Roy Araiza, Travis Russell, and Mark Tomforde. ``A universal representation for quantum commuting correlations''. Ann. Henri Poinc. 23, 4489–4520 (2022). arXiv:2102.05827.
https://doi.org/10.1007/s00023-022-01197-7
arXiv:2102.05827
[95] I. Pitowsky. ``Quantum probability – quantum logic''. Volume 321 of Lect.Notes Phys. Springer. (1989).
https://doi.org/10.1007/BFb0021186
[96] Dan Geiger, Christopher Meek, Bernd Sturmfels, et al. ``On the toric algebra of graphical models''. Ann. Statist. 34, 1463–1492 (2006). arXiv:math/0608054.
https://doi.org/10.1214/009053606000000263
arXiv:math/0608054
Cited by
[1] Antoni Mikos-Nuszkiewicz and Jędrzej Kaniewski, "Extremal points of the quantum set in the CHSH scenario: conjectured analytical solution", arXiv:2302.10658, (2023).
[2] José Jesus and Emmanuel Zambrini Cruzeiro, "Tight Bell inequalities from polytope slices", arXiv:2212.03212, (2022).
[3] Rafael Wagner, Rui Soares Barbosa, and Ernesto F. Galvão, "Inequalities witnessing coherence, nonlocality, and contextuality", arXiv:2209.02670, (2022).
[4] Lina Vandré and Marcelo Terra Cunha, "Quantum sets of the multicolored-graph approach to contextuality", Physical Review A 106 6, 062210 (2022).
The above citations are from SAO/NASA ADS (last updated successfully 2023-03-22 14:01:01). The list may be incomplete as not all publishers provide suitable and complete citation data.
On Crossref's cited-by service no data on citing works was found (last attempt 2023-03-22 14:00:59).
This Paper is published in Quantum under the Creative Commons Attribution 4.0 International (CC BY 4.0) license. Copyright remains with the original copyright holders such as the authors or their institutions.
- SEO Powered Content & PR Distribution. Get Amplified Today.
- Platoblockchain. Web3 Metaverse Intelligence. Knowledge Amplified. Access Here.
- Source: https://quantum-journal.org/papers/q-2023-03-16-947/
- :is
- ][p
- 1
- 1.3
- 10
- 100
- 11
- 110
- 1985
- 1998
- 1999
- 2001
- 2011
- 2012
- 2014
- 2016
- 2017
- 2018
- 2019
- 2020
- 2021
- 2022
- 2023
- 28
- 39
- 67
- 7
- 70
- 77
- 8
- 84
- 9
- a
- About
- above
- ABSTRACT
- AC
- access
- accessed
- achieved
- advances
- affiliations
- AL
- Alexander
- algorithms
- All
- amsterdam
- analysis
- Analytical
- and
- Andrew
- annual
- Application
- applications
- approach
- ARE
- Argentina
- Art
- AS
- aspect
- At
- atom
- author
- authors
- available
- average
- ball
- based
- Basel
- basis
- BE
- Bell
- Berkeley
- berlin
- between
- body
- boris
- Break
- by
- CA
- CAD
- california
- CAN
- case
- Certified
- Charles
- chen
- Choose
- Christopher
- Claus
- closed
- Coin
- comment
- Commons
- commuting
- COMP
- complete
- completion
- complex
- complexity
- comprehensive
- condition
- Conference
- conjecture
- contains
- Convex
- coordinate
- copyright
- Correlation
- course
- cryptographic
- cryptography
- Current
- cycle
- cycles
- Daniel
- data
- David
- deliver
- Den
- description
- Design
- detailed
- details
- Devices
- Dimension
- dimensions
- discuss
- Distant
- distribution
- domains
- dynamics
- e
- each
- edition
- essentially
- establish
- Ether (ETH)
- exactly
- experiment
- exposed
- extreme
- faces
- field
- Fields
- First
- fixed
- For
- form
- Forum
- found
- Fourth
- from
- Frontiers
- function
- functions
- fundamental
- Games
- General
- generated
- generates
- geometry
- Gilles
- GMS
- goal
- graduate
- graphs
- Group’s
- guide
- Hall
- Hang
- harvard
- henry
- hierarchy
- High
- holders
- holds
- How
- HTML
- http
- HTTPS
- i
- identifying
- IEEE
- image
- implementation
- important
- in
- inequalities
- Inequality
- info
- information
- Institute
- institutions
- integral
- interesting
- International
- ITS
- JavaScript
- Jian-Wei Pan
- John
- Johnson
- journal
- Key
- known
- larger
- Last
- Leave
- Library
- License
- links
- List
- little
- local
- LOOKS
- M2
- Main
- many
- mark
- matching
- math
- mathematical
- mathematics
- Matrix
- max-width
- measurements
- measures
- methods
- Michael
- minimal
- mixed
- model
- models
- Modern
- moment
- Month
- Moon
- most
- Nature
- necessary
- New
- Nicolas
- normal
- Notes
- numbers
- of
- on
- ONE
- open
- operator
- operators
- optics
- optimization
- Orange
- original
- overview
- PAN
- Paper
- Paradox
- particular
- parties
- Patches
- perspectives
- Peter
- phase
- philadelphia
- Philippe
- Photons
- Physics
- Pierre
- plato
- Plato Data Intelligence
- PlatoData
- points
- polar
- position
- positive
- possible
- Practical
- Practical Applications
- principle
- principles
- privacy
- probability
- Problem
- problems
- Proceedings
- Programming
- Programs
- Progress
- projections
- proofs
- property
- protocols
- provide
- public
- Public Key
- published
- publisher
- publishers
- Publishing
- Quantum
- quantum information
- Quantum optics
- quantum systems
- Quick
- Rafael
- random
- Rates
- real
- Reality
- realization
- realized
- recent
- references
- regions
- relations
- relationship
- remains
- representation
- research
- resulting
- Results
- review
- Richard
- s
- same
- satisfied
- scenario
- scenarios
- SCI
- Science
- SCIENCES
- Second
- secure
- security
- Series
- set
- Sets
- several
- siam
- Simon
- since
- site
- Soccer
- Software
- solution
- son
- South
- Space
- spaces
- specific
- specified
- spins
- State
- States
- Stephen
- street
- structure
- studies
- Successfully
- such
- sufficient
- suitable
- supplement
- Surface
- Symposium
- system
- Systems
- Terra
- test
- Testing
- that
- The
- their
- Them
- theoretical
- These
- three
- three-dimensional
- Tim
- Timo
- Title
- to
- today
- Transactions
- Transformation
- UN
- under
- understanding
- unique
- Universal
- university
- University of California
- updated
- URL
- users
- via
- VIOLATION
- volume
- volumes
- vw
- W
- What
- What is
- which
- Wikipedia
- with
- witness
- witnessing
- Wolf
- Work
- works
- Wright
- wu
- year
- zephyrnet